GSEB Solutions Class 10 Maths Chapter 8 Introduction to Trigonometry Ex 8.2

Gujarat Board GSEB Solutions Class 10 Maths Chapter 8 Introduction to Trigonometry Ex 8.2 Textbook Questions and Answers.

Gujarat Board Textbook Solutions Class 10 Maths Chapter 8 Introduction to Trigonometry Ex 8.2

Question 1.
(i) sin 60° cos 30° + sin 30° cos 60°
= \(\frac{\sqrt{3}}{2} \times \frac{\sqrt{3}}{2}+\frac{1}{2} \times \frac{1}{2}=\frac{3}{4}+\frac{1}{4}\)
= \(\frac {3 + 1}{4}\) = \(\frac {4}{4}\) = 1

(ii) 2 tan² 45° + cos² 30° – sin² 60°
= 2(1)² + \(\left(\frac{\sqrt{3}}{2}\right)^{2}-\left(\frac{\sqrt{3}}{2}\right)^{2}\)
= 2 + \(\frac{3}{4}-\frac{3}{4}\) = 2

(iii) \(\frac {cos 45°}{sec 30°+ cosec 30°}\)



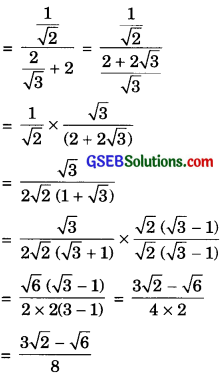
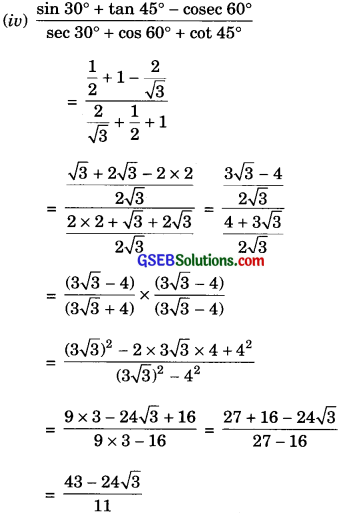
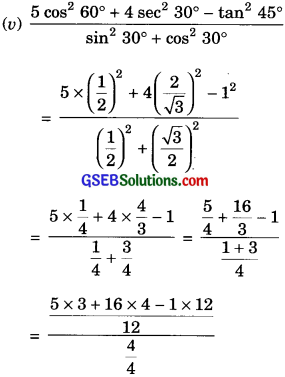
= \(\frac{15+64-2}{12}\) = \(\frac{79-2}{12}=\frac{77}{12}\)

Question 2.
Choose the correct option and justifr your choice:
(i) \(\frac{2 \tan 30^{\circ}}{1+\tan ^{2} 30^{\circ}}\)
(a) sin 60°
(b) cos 60°
(c) tan 60°
(d) sin 30°

(ii) \(\frac{1-\tan ^{2} 45^{\circ}}{1+\tan ^{2} 45^{\circ}}\)
(a) tan 90°
(b) 1
(c) sin 45°
(d) O

(iii) sin 2A = 2 sin A is true when A =
(a) 0°
(b) 30°
(c) 45°
(d) 60°

(iv) \(\frac{2 \tan 30^{\circ}}{1-\tan ^{2} 30^{\circ}}\)
(a) cos 60°
(b) sin 60°
(c) tan 60°
(d) sin 30°
Solution:

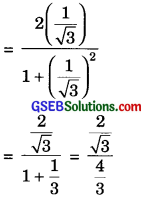
= \(\frac{2}{\sqrt{3}} \times \frac{3}{4}=\frac{\sqrt{3}}{2}\) = sin 60°
Hence, correct option is (a) sin 60°.

(ii) \(\frac{1-\tan ^{2} 45^{\circ}}{1+\tan ^{2} 45^{\circ}}\)
= \(\frac{1-(1)^{2}}{1+1^{2}}\)= \(\frac{1-1}{2}\) = 0
Hence correct option is (d).

(iii) When 0°
sin 2A = sin 2(0°) = sin 0° = 0
2 sin 2 sin (0°) = 2(0) = 0
∴ sin 2A = 2 sin A
Hence, the correct option is (a) 0°.

(iv) \(\frac{2}{\sqrt{3}} \times \frac{3}{2}\) = \(\sqrt{3}\)

= tan 60°
Hence, the correct option is (c) tan 60°.

Question 3.
If tan (A + B) = \(\sqrt{3}\) and tan (A – B) = \(\frac{1}{\sqrt{3}}\)0° < A + B ≤ 90°, A > B, find A and B.
Solution:
We have
tan(A + B) = \(\sqrt{3}\)
⇒ tan (A + B) = tan 60°
⇒ A + B = 60° ……….(1)
tan (A – B) =
⇒ tan(A – B) = tan 30°
⇒ A – B = 30° …………(2)
From eqn. (1) and (2), we get
2A = 90°
A = 45°
From (1), A + B = 90°
⇒ 45° + B = 90°
⇒ B = 90° – 45°
⇒ B = 45°

Question 4.
State whether the following are true or false Justify your answer.
(i) sin (A + B) = sin A + sin B.
(ii) The value of sin θ increases as θ increase
(iii) The value of cos θ increases as θ increase
(iv) sin θ = cos θ for all values of θ.
(v) cot θ is not defined for A = 0°.
Solution:
(i) False
We have
sin(A + B) = sin A + sin B
Let A = 30°,and B = 60°
then LHS = sin (A + B)
= sin (30° + 60°)
= sin 90° = 1
RHS = sin A + sin B
= sin 30° + sin 60°
= \(\frac{1}{2}+\frac{\sqrt{3}}{2}\)
= \(\frac{1+\sqrt{3}}{2}=\frac{\sqrt{3}+1}{2}\)
LHS ≠ RHS

(ii) True
Value of sin θ, increases as θ increases.
(iii) False
Value of cos θ decreases as θ increases.
(iv) False
sin θ = cos θ is not true to all values of θ, as
we take θ = 30°
LHS = sin 30° = and RHS = cos 30° = \(\frac{\sqrt{3}}{2}\)
Thus, sin 30° ≠ cos 30°
(v) True
cos A = \(\frac{\cos A}{\sin A}=\frac{\cos 0^{\circ}}{\sin 0^{\circ}}=\frac{1}{0}\) Not defined