This GSEB Class 7 Maths Notes Chapter 1 Integers covers all the important topics and concepts as mentioned in the chapter.
Integers Class 7 GSEB Notes
Brahmagupta:
Brahmagupta was the Indian Mathematician who defined zero. He set the rules for its computation. These rules further led to make he mathematical problems real and easily solvable.
There are numerous area in the field of mathematics where the contribution of Indian mathematician has been immense. It includes the discovery of zero, the rules of working with negative numbers and a system of expressing all the numbers using only ten symbols.
- Integers: The integers are the numbers used for counting forward as well as backward. The use of integers in our real life makes them important in mathematics too. Integers help in computing the efficiency in positions, to calculate how more or less measures to be taken for achieving better results and many more.
- Natural Numbers: As we know that numbers used for counting are called Natural numbers. (N = 1, 2, 3, 4, 5, ……..)
- Whole numbers.: All natural numbers along with 0 (Zero) are called whole numbers (W = 0, 1,2, 3,4, 5…).
But these numbers cannot help us to solve all our daily life problems. Therefore we shall study integers, which is a collection of whole numbers and the negatives of natural numbers.
………., -5, -4, -3, -2, -1, 0, 1, 2, 3, 4, 5 ……….. 1, 2, 3, 4, 5,…….. are positive integers.
– 1, – 2, – 3, – 4, – 5, ……….. are negative integers.
0 (Zero) is an integer which is neither positive nor negative.
Representation of Integers on a Number line
Draw a line. Mark a point 0 on the line. Label it as O. Now mark some more points at equal distances on the right as well as the left of 0. Label the points on the right side of 0 as 1, 2, 3………. and label the points on the left side as – 1, -2,-3, ……….. as shown below:
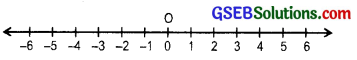
The arrowheads on both the sides of the number line indicate the continuation of integers infinitely on each side.
Absolute Value of an Integer
The absolute value of an integer ‘a’ is the number value of ‘a’ regardless of its sign. It is denoted by |a|, called the modulus of a
for example
- | 6 | = 6 and | – 6 | = 6
- | – 8 | = 8 and | 8 | = 8.
![]()
Four Fundamental Operations
Addition of Integers:
1. Addition of two integers having same signs:
- Step 1. Add the values regardless of their sign.
- Step 2. Write the sum with sign of both the integers.
2. Addition of two integers having different signs:
- Step 1. Find the difference between the values regardless of their sign.
- Step 2. Write the difference with the sign of the integer having greater value.
3. Addition of three or more integers.
- Step 1. Add all positive integers together. Add their values regardless of their signs and write the sum with positive sign.
- Step 2. Add all negative integers together. Add their values regardless of their signs and write the sum with negative sign.
- Step 3. Find the difference between their numerical values regardless of their signs and write the difference with the sign of greater value.
Properties of Addition of Integers
1. Closure property. Integers are closed for addition. The sum of two integers is also an integer, i.e. if a and b are integers then a + b is also an integer.
For example
3 + (- 5) = – 2, (- 6) + (9) = 3, 8 + 7 = 15
2. Commutative property. For all integers a and b
a + b = b + a
For example:
6 + 9 = 9 + 6 = 15
3. Associative property. For all integers a, b and c.
a + (b + c) = (a + b) + c
For example:
(-3) + (6 + 9) | [(-3) + 6]+ 9
= (- 3) + (15) | = (3) + 9
= 12 | = 12
(- 3) + (6 + 9) = [(- 3) + 6] + 9
4. Additive identity: The integer ‘0’ is such that a + 0 = 0 + a = a ‘0’ is called the additive identity of integers.
5. Additive Inverse: For any integer a, we have (- a) + a = 0 = a + (- a)
The negative of an integer a is (- a) and the sum of an integer and its negative is ‘0’
∴ Additive inverse of a is (- a)
Similarly additive inverse of (- a) is – (- a) = a
Subtraction of Integers: Subtracting an integer from another integer is same as adding first integer with additive inverse of second integer. In other words, if a and b are two integers then a – (+ b) = a + (- b).
Properties of Subtraction of Integers
1. Closure property: The difference of two integers is an integer i.e. if a and b are any two integers then (a – b) is always an integer.
2. Subtraction of integers is not commutative.
For Example:
(4 – 9) | (9 – 4)
= – 5 | 5
∴ 4 – 9 ≠ 9 – 4
3. Subtraction of integers is not associative
For Example:
[9 – (- 2)] – 1 | 9 – [(- 2) – 1]
= [9 + 2] – 1 | = 9 – [- 3]
= 11 – 1 | = 9 + 3
= 10 | = 12
∴ [9 – (- 2)] – 1 ≠ 9 – [(- 2) – 1]
4. For every integer a, a – 0 = a ≠ 0 – a
Multiplication of Integers
Multiplication is Repeated Addition:
Let a and b are positive integers then a × b defined as addition of a to b times or addition of b to a times.
For Example: 5 × 3 = 5 × 5 × 5 = 15
Or
3 × 5 = 3 × 3 × 3 × 3 × 3 = 15
Multiplication of integers with same sign:
- Step 1. Multiply the numbers regardless of their sign.
- Step 2. Write the product with a positive sign.
Multiplication of integers with different sign:
- Step 1. Multiply the numbers regardless of their sign.
- Step 2. Write the product with a negative sign.
![]()
Product of three or more Negative Integers
To find the product of three or more negative integers we can simply take two integers at a time and follow the rules as for the multiplication of two integers.
(-a) × (-b) × (-c) = [(-a) × (-b)] × (-c)
= (a × b) × (-c)
= – (a × b × c)
Properties of Multiplication of integers
1. Closure Property. If a and b are two integers then a × b is also an integer.
For example: – 6 and 8 are integers then -6 × 8 = -48 is also an integer.
2. Commutative property. If a and b are two integers. Then ax b is same as b × a
i. e. a × b = b × a.
For example: 3 × 4 = 4 × 3 = 12
3. Associative property for multiplication. If a, b and c are three integers then
a × (b × c) = (a × b) × c or (a × b) × c = (a × c) × b
For example: 3 × (5 × 8) = (3 × 5) × 8
= 120
4. Distributive property:
(a) Distributive property of multiplication over addition. If a, b and c are three integers then
a × (b + c)= (a × b) + (a × c)
For example: 9 × (8 + 2) = (9 × 8) + (9 × 2) = 72 + 18 = 90.
(b) Distributive property of multiplication over subtraction. If a, b and c are three integers then
a × (b – c)= (a × b) – (a × c)
For example: 8 × (9 – 4) = (8 × 9) – (8 × 4) = 72 – 32 = 40.
5. Multiplicative property of zero
For any Integer a we have
a × 0 = 0 × a = 0
For example 9 × 0 = 0 × 9 = 0
6. Multiplicative Identity
For any integer a we have
a × 1 = 1 × a = a
For example:
8 × 1 = 1 × 8 = 8
For easier multiplication we can use commutative associative and distributive property of integers
For example:
30 × 5 + 30 × -2 = 30 × (5 – 2)
= 30 × 3 = 90.
Division of two Integers
1. Division of integers with same signs
- Step 1. Divide the numbers regardless of their signs.
- Step 2. Write the quotient with a positive sign.
2. Division of integers with different signs:
- Step 1. Divide the numbers regardless of their sign.
- Step 2. Write the quotient with a negative sign.
Properties of Division of Integers
1. When an integer is divided by another, the quotient need not be an integer.
For example:
- 3 and 8 are two integers, but 3 ÷ 8 is not an integer i.e. \(\frac{3}{8}\) is not an integer.
- -3 and 7 are two integers but (-8) ÷ 9 is not an integer i.e. \(\frac{-8}{9}\) is not an integer.
2. For every non-zero integer a, we have a ÷ a = 1.
For example:
- (+9) ÷ (+9) = 1
- (-6) ÷ (-6) = 1
3. For every non-zero integer a, we have 0 ÷ a = 0
For example
- 0 ÷ (+8) = 0
- 0 ÷ (-3) = 0
4. For non-zero integers a and b, where a ≠ b we have a ÷ b ≠ b ÷ a (i.e. commutative propoerty does not hold)
For example: 10 ÷ 5 = 2 but 5 ÷ 10 = \(\frac{1}{2}\)
5. For non-zero integer a, b and c where a ≠ b ≠ c we have (a ÷ b) ÷ c ≠ a ÷ (b ÷ c) (i.e. Associative property does not hold).
Even and Odd Integers
Even Integers: All the integers, which are exactly divisible by 2 are called even integers.
…….., -8, -6, -4,- -2, 0, 2, 4, 6, 8……….. are some even integers.
Odd Integers: All the integers which are not exactly divisible by 2 are called odd integers. ……….-7, -5, -3, -1, 1, 3, 5, 7,…………. are some odd Integers.