This GSEB Class 6 Maths Notes Chapter 1 Knowing Our Numbers covers all the important topics and concepts as mentioned in the chapter.
Knowing Our Numbers Class 6 GSEB Notes
Introduction:
In our previous classes, we have worked with numbers. We have added, subtracted, multiplied and divided them. We also looked for patterns in number sequences. In this chapter we shall move forward on such interesting things with a bit of review and revision as well.
Natural Numbers:
We are using numbers 1, 2, 3, 4, 5, etc. to count and calculate. These counting numbers are called natural numbers.
Facts About Natural Numbers
- Smallest or first Natural Number is ‘ 1’.
- By adding 1 to any natural number we can get next natural number.
- There is no largest natural number.
- Set of natural numbers is represented by ‘N’.
N={1, 2,3,4, }
Digits:
To represent any number, we use ten symbols 0, 1, 2, 3, 4, 5, 6, 7, 8 and 9. These ten symbols are called digits.
Comparing Numbers:
To compare two numbers, we use the following rules :
Rule 1:
When two numbers have different number of digits, the number having more number of digits is greater than the number having less number of digits.
Rule 2:
(a) When two numbers have same number of digits, the number having greater first left most digit is greater.
(b) If their first left most digit is same then the number having greater second left most digit is greater.
(c) Continue the process till you get unequal digit at the corresponding place.
(d) Apply the same process while comparing more than two numbers.
![]()
Ascending (Increasing) Order:
Ascending order means arrangement of the numbers from smallest to greatest.
Descending (Decreasing) Order:
Descending order means arrangement of numbers from greatest to smallest.
Some Important Observations:
- Greatest single digit number + 1 = Smallest 2 – digit number
- Greatest 2 – digit number + 1 = Smallest 3 – digit number
- Greatest 3 – digit number + 1 = Smallest 4 – digit number and so on.
Place Value and Face Value:
The place value of a digit depends on its position, whereas the face value does not depend on its position. For example in the number 6598 the face value of 8 is 8. Similarly the face value of 9, 5 and 6 are also 9, 5 and 6 respectively.
However when we are concerned with place value.
- The digit 8 has the place value = 8 × 1 = 8 (8 lies at units place)
- The digit 9 has the place value = 9 × 10 = 90 (9 lies at tens place)
- The digit 5 has the place value = 5 × 100 = 500 (5 lies at hundreds place)
- The digit 6 has the place value = 6 × 1000 = 6000 (6 lies at thousands place)
In expanded form 9678 will be written as 6598 = 6 × 1000 + 5 × 100 + 9 × 10 + 8 × 1
- It is evident from this that a number is the sum of the place values of all its digits.
- Place value of a digit – Face value × Position value
It is to be noted that position values of units, tens, hundreds, thousands, ten thousands, lakhs and so on are respectively 1,10, 100, 1000, 10000, 100000, and so on.
Place value of 0 is 0 itself, where ever it may be.
Indian System of Numeration
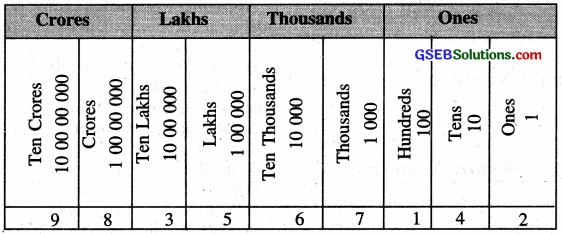
Reading and Writing Numbers in Indian System of Numeration
In order to read numbers in the Indian system of Numeration, we make groups (periods) of place values like : ‘Ones’, Thousands, Lakhs, Crores etc, separated by commas.
- The First three digits from the right of a number make unit period (or unit group).
- The next two digits from the right make thousands period.
- The next two digits from right make Lakhs period.
- The next two digits from right make crores period and so on
The digits in the same group or period are read together and the name of the period (except units) is read along with them.
While reading a numeral, all the digits in the same period are read together and the name of the period (except the ones) is read along with them. Example : We read 983567142 as ninety eight crore; thirty five lakh, sixty seven thousand, one hundred forty two.
![]()
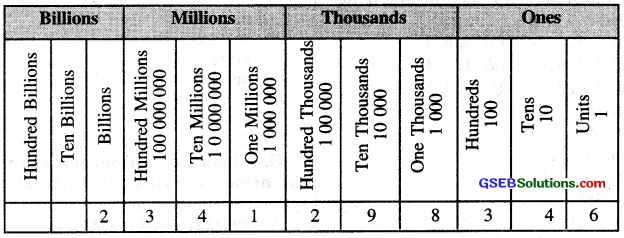
International System of Numeration:
In this system a number is split up into groups or periods. Starting from extreme right the number is split up into groups of three each. These groups are called Ones, Thousands, Millions and Billions. These groups are further sub-divided as follow :
In this system ones, tens, thousands, millions and billions are used. Commas are used to mark thousands and millions. The first comma marks Thousands, second and the next comma marks Millions.
Example: The number 2, 341, 298, 346 is read as Two billion three hundred forty one million two hundred ninety eight thousand three hundred forty six.
International Place – Value Chart
Numbers in Length, Weight and Capacity:
(Use Numbers in Practical Life)
1. Length:
We express the length of a pencil or paper in centimetres. Whereas we express the thickness of a paper clip in centimetres. We use smaller units i.e. millimetre. On the other hand to measure height of a building. We need bigger units that is metre. And we need bigger units to express distance between two cities, which is kilometre.
Remember:
- 10 millimetres = 1 centimetre
- 1 metre =100 centimetre
= 1000 millimetre - 1 kilometre = 1000 metres
= 1000000 millimetres
2. Weight:
We buy things like turmeric, ginger and garlic in grams, whereas to buy things like flour, potatoes and rice we use bigger units like Kilograms. But medicine we consume is in milligrams.
- 1 kilogram = 1000 gram
- 1 gram = 1000 miligram
3. Capacity:
We drink water in a glass, it is approximately 250 to 300 ml. When we fill a bucket with water to have bath, it is nearly 20 to 25 litre; a bigger unit.
1 litre = 1000 millilitre.
Remember:
kilo shows 1000 times larger, centi shows 100 times smaller. Thus, 1 kilometer = 1000 metres, 1 metre = 100 centimetres or 1000 millimetres etc.
Estimation and Approximation Roun-ding Numbers
A large number may be rounded off to the nearest 10, 100,1000, 10,000, 100,000 etc.
Nearest 10:
(1) While rounding off to the nearest ten if the digit in the unit’s place is between 0-4 i.e., < 5 then the unit’s digit is replaced by 0.
(2) If the digit in the unit place is 5 to 9
i. e., > or = 5 but < 10 , then the unit’s place is replaced by ‘0’ and the ten’s place increased by 1.
Example :
6,84,523 → 684520
3 < 5.
Nearest 100:
- While rounding off to the nearest hundred, if the digits in the ten’s place is between 0-4 i.e. < 5 then the ten’s place and all the following places are replaced by ‘0’.
- If the digit in the ten’s place is 5 to 9
i. e. > or = 5 but < 10 then the ten’s place and all the following places are replaced by ‘0’ and the hundred place is increased by ‘1’.
Example :
(a) 58,553 → 58,600
∵ Ten’s digit is 5.
(b) 2,86,789 → 2,86,800
∵ 8 > 5.
Nearest 1000:
- While rounding off to nearest thousand, if the digit in the hundred’s place is betwen 0-4 i.e. < 5, then the hundred’s place and all the following places are replaced by ‘0’.
- If the digit in the hundreds place is = to or > 5 then the hundred’s place and all the following places are replaced by ‘0’ and the thousand’s place is increased by 1.
Example :
(a) 4,35,172 → 4,35,000
∵ 1 < 5
(b) 68,58,942 → 68,59,000
∵ 9 > 5.
![]()
Use of Brackets:
Brackets are symbols used in pairs to group things together and write statements in explicit form
Most commonly used brackets are ():
- Parentheses or common brackets { }:
- Curly Brackets [ ]:
- Square Brackets or Box Brackets.
- In this section, we shall learn about the use of parentheses only.
Expanding Brackets
To expand brackets, we need to use distributive law. Distributive law states that a number outside a bracket performs the same operation with each term inside the bracket as follow :
a (b + c) = ab + ac a (b – c) – ab – ac.
Roman Numerals
There are 7 basic symbols in Roman system which are used to represent different numbers.
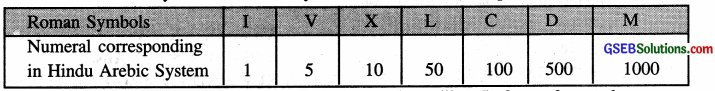
This system of Numeration is still used in many p aces like : In front of your class rooms (Class VI etc.), numbers on clock faces, parts of books, to denote historical events such as : world war I, world war II, etc.
Corresponding to ‘0’ of Hindu Arabic system, there is no symbol for 0 (Zero) in Roman Numeration system.
Using these 7 symbols, we can write number by following certain rules which are given below :
Rule 1:
If a symbol is repeated its value, it is added as many times as it occours. For Example :
II = 1 + 1 = 2
XXX = 10 + 10 + 10 = 30
CC = 100 + 100 = 200
It must be noted that symbol I, X, C, M never repeated more than three times, and V, L, D are never repeated.
Rule 2:
Any smaller Roman numeral that comes after a larger numeral is added to it.
For Example :
VII = 5 + 1 + 1 = 7 XVII = 10 + 5 + 1 + 1 = 17 LXXV = 50 + 10 + 10 + 5 = 75
Rule 3:
Any smaller Roman numeral that comes before a larger numeral is subtracted from it.
For Example :
IV = 5 – 1 = 4 = 10 – 1 = 9 = 50 – 10 = 40 = 100 – 10 = 90
Rule 4:
The symbols V, L and D are never written to the left of a larger value symbol, i.e. V, L, D are never subtracted.
Rule 5:
If a smaller Roman numeral comes between two larger numerals then the smaller numeral is subtracted from the larger numeral following it:
For Example :
XIX = X + IX = 10 + (10 – 1) = 19 LXIV = L + X + IV = 50 + 10 + (5 – 1) = 64
Rule 6:
If a bar is placed over a numeral then it is multiplied by 1000.
For Example :
V = 5000, X = 10000