Gujarat Board Statistics Class 12 GSEB Solutions Part 2 Chapter 1 Probability Ex 1.2 Textbook Exercise Questions and Answers.
Gujarat Board Textbook Solutions Class 12 Statistics Part 2 Chapter 1 Probability Ex 1.2
Question 1.
A balanced coin is tossed three times. Find the probability of the following events:
(1) Getting all three heads
(2) Not getting a single head
(3) Getting at least one head
(4) Getting more than one head
(5) Getting at the most one head
(6) Getting less than two heads
(7) Getting head and tail alternately
(8) Getting more number of heads than tails.
Answer:
A balanced coin is tossed three times. So the total number of primary outcomes of its sample space is = n = 23 = 8.
U = {HHH, HHT, HTH, THH, THT, HTT, TTH, TTT}
(1) A = Event of getting all three heads.
A = {HHH}
∴ Favourable outcomes for the event A is m = 1.
Hence, P(A) = \(\frac{m}{n}\) = \(\frac{1}{8}\)
(2) B = Event of not getting a single head
B = {TTT}
∴ Favourable outcomes for the event B is m = 1.
Hence, P(B) = \(\frac{m}{n}\) = \(\frac{1}{8}\)

( 3 ) B’ = Event of getting at least one head,
i. e., getting all three tails
∴ P(B’) = 1 – P(B)
= 1 – \(\frac{1}{8}\) = \(\frac{7}{8}\)
(4) C = Event of getting more than one head.
C = {THT, HTT, TTH, TTT}
∴ Favourable outcomes for the event C is m = 4.
Hence, P(C) = \(\frac{m}{n}\) = \(\frac{1}{8}\) = \(\frac{1}{2}\)
(5) D = Event of getting at the most one head, i.e., 0 or 1 head
D = {THT, HTT, TTH, TTT}
∴ Favourable outcomes for the event D is m = 4.
Hence, P(D) = \(\frac{m}{n}\) = \(\frac{4}{8}\) = \(\frac{1}{2}\)
(6) E = Event of getting less than two heads,
i. e., 0 or 1 head = D
∴ P(E) = P(D) = \(\frac{1}{2}\)
(7) F = Event of getting head and tail alternatively
F = {HTH, THT}
∴ Favourable outcomes for the event F is m = 2.
Hence, P(F) = \(\frac{m}{n}\) = \(\frac{2}{8}\) = \(\frac{1}{4}\)
(8) G = Event of getting more number of heads than tails.
G = {THT, HTT, TTH, TTT}
∴ Favourable outcomes for the event G is m = 4.
Hence, P(G) = \(\frac{m}{n}\) = \(\frac{4}{8}\) = \(\frac{1}{2}\)

Question 2.
Two balanced dice are thrown simultaneously. Find the probability of the following events:
(1) The sum of numbers on the dice is 6.
(2) The sum of numbers on the dice is not more than 10.
(3) The sum of numbers on the dice is a multiple of 3.
(4) The product of numbers on the dice is 12.
Answer:
Two balanced dice are thrown simultaneously. So the sample space for this random experiment Is expressed as follows:
U ((1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6), (2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, 6), (3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6), (4: 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6), (5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6), (6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}
∴ Total number of primary outcomes of U is
n = 36
(1) A = Event that the sum of numbers on the dice is 6.
A = {( 1, 5), (2. 4), (3, 3), (4, 2). (5. 1))
∴ Favourable outcomes for the event A is m = 5.
Hence, P(A) = \(\frac{m}{n}\) = \(\frac{5}{36}\)
(2) B = Event that the sum of numbers on the dice is more than 10.
∴ B’ = Event that the sum of numbers on the dice is not more than 10.
B = {(5, 6), (6, 5), (6, 6)}
∴ Favourable outcomes for the event B is m = 3.
Hence P(B) = \(\frac{m}{n}\) = \(\frac{3}{36}\) = \(\frac{1}{12}\)
∴ P(B’) = 1 – P(B)
= 1 – \(\frac{1}{12}\) = \(\frac{11}{12}\)
(3) C = Event that the sum of numbers on the dice is a multiple of 3, i.e., 3, 6, 9 or 12
C = {(1, 2), (2, 1), (1, 5), (2, 4), (3, 3), (4, 2), (5, 1), (3, 6), (4, 5), (5, 4), (6, 3), (6, 6)}
∴ Favourable outcomes for the event C is m = 12
Hence, P(C) = \(\frac{m}{n}\) = \(\frac{12}{36}\) = \(\frac{1}{3}\)
(4) D = Event that the product of the numbers on the dice is 12
D = {(2, 6), (3, 4), (4, 3), (6, 2)}
∴ Favourable outcomes for the event D is m = 4
Hence, P(D) = \(\frac{m}{n}\) = \(\frac{4}{36}\) = \(\frac{1}{9}\)
Question 3.
One family is randomly selected from the families having two children. Find the probability that
(1) One child is a girl and one child is a boy.
(2) At least one child is a girl among the two children of the selected family.
(Note: Assume that the chance of the child being a boy or girl is same.)
Answer:
We take, B = Boy, G = Girl
∴ The sample space for the families having two children is expressed as follows:
U = {BB, BG, GB, GG}
Now, the total number of primary outcomes of the sample space of selecting a family at random is n = 4C1 = 4.
(1) A = Event that one child is a girl and one child is a boy.
= {BG, GB}
∴ Favourable outcomes for the event A is m = 2.
Hence, P(A) = \(\frac{m}{n}\) = \(\frac{2}{4}\) = \(\frac{1}{2}\)
(2) B = Event that at least one child is a girl among two children of the selected family.
= {GB, BG, GG}
∴ Favourable outcomes for the event B is m = 3.
Hence, P(B) = \(\frac{m}{n}\) = \(\frac{3}{4}\)

Question 4.
One number is selected at random from the first 100 natural numbers. Find the probability that this number is divisible by 7.
Answer:
Here, U = {1, 2, 3, …, 100}
One number is selected at random.
∴ Total number of primary outcomes
n = 100C1 = 100
A = Event that the number selected is divisible by 7.
A = {7, 14, 21, 28, …, 91, 98}
∴ Favourable outcomes for the event A is m = 14.
Hence, P(A) = \(\frac{m}{n}\) = \(\frac{14}{100}\) = \(\frac{7}{50}\)
Question 5.
The sample space for a random experiment of selecting numbers is U = (1, 2, 3, …. 120} and all the outcomes in the sample space are equiprobable. Find the probability that the number selected is:
(1) a multiple of 3
(2) not a multiple of 3
(3) a multiple of 4
(4) not a multiple of 4
(5) a multiple of both 3 and 4
Answer:
Here, U = {1, 2, 3, …, 120} ‘
A number is selected at random.
∴ Total number of primary outcomes is n = 120C1 = 120.
(1) A = Event that the number selected is a multiple of 3.
= {3, 6, 9, 12, …, 117, 120}
∴ Favourable outcomes for the event A is m = 40.
Hence, P(A) = \(\frac{m}{n}\) = \(\frac{40}{120}\) = \(\frac{1}{3}\)
(2) A’ = Event that the number selected is not a multiple of 3.
∴ P(A’) = 1 – P(A)
= 1 – \(\frac{1}{3}\) = \(\frac{2}{3}\)
(3) B = Event that the number selected is a multiple of 4.
= {4, 8, 12, 16, …, 116, 120}
∴ Favourable outcomes for the event B is m = 30.
Hence, P(B) = \(\frac{m}{n}\) = \(\frac{30}{120}\) = \(\frac{1}{4}\)
(4) B’ = Event that the selected number is not a multiple of 4.
∴ P(B’) = 1 – P(B)
= 1 – \(\frac{1}{4}\) = \(\frac{3}{4}\)
(5) A ∩ B = Event that the number selected is a multiple of both 3 and 4, i.e., a multiple of 12.
∴ A ∩ B = {12, 24, 36, 48, …, 108, 120}
∴ Favourable outcomes for the event A ∩ B is m = 10.
Hence, P(A ∩ B) = \(\frac{m}{n}\) = \(\frac{10}{20}\) = \(\frac{1}{12}\)

Question 6.
Find the probability of getting R in the first place and M in the last place when all the letters of the word RANDOM are arranged in all possible ways.
Answer:
Here, there are 6 letters R, A, N, D, O, M in the word RANDOM.
∴ The total number of ways of arranging these six letters is,
n = 6P6 = 6! = 6 × 5 × 4 × 3 × 2 × 1 = 720.
A = Event that getting R in the first place and M in the last place.
∴ Favourable outcomes for the event A are obtained as follows:
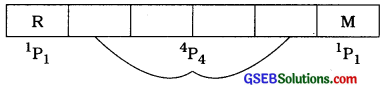
∴ m = 1P1 × 4P4 × 1P1
= 1! × 4! × 1!
= 1 × 24 ×1 = 24
Hence p(A) = \(\frac{m}{n}\) = \(\frac{24}{720}\) = \(\frac{1}{30}\)
Question 7.
Find the probability of getting vowels in the first, third and sixth place when all the letters of the word, ORANGE are arranged in nil possible ways.
Answer:
Here, there are total 6 letters O, R, A, N, G, E in the word ORANGE.
And there are 3 vovels O, A, E
∴ The total number of ways of arranging these six letters,
n = 6P6 = 6! = 6 × 5 × 4 × 3 × 2 × 1 = 720
A = Event of getting vowels in the first, third and sixth place.
∴ The favourable outcomes for the event A are obtained as follows:
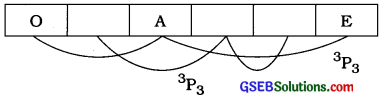
∴ m = 3P3 × 3P3 = 3! × 3!
= 6 × 6 = 36
Hence, P(A) = \(\frac{m}{n}\) = \(\frac{36}{720}\) = \(\frac{1}{20}\)
Question 8.
Five members of a family, husband, wife and three children are randomly arranged in a row for a family photograph. Find the probability that the husband and wife are seated next to each other.
Answer:
Five members of a family can be arranged in,
n = 5P5 = 5! = 5 × 4 × 3 × 2 × 1 = 120 ways.
A = Event that the husband and wife are seated next to each other.
∴ The favourable outcomes for the event A are obtained as follows:
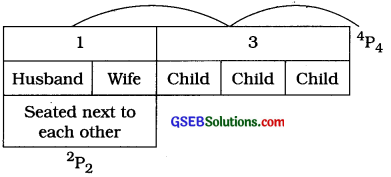
∴ m = 2P2 × 4P4
= 2! × 4!
= 2 × 24
= 48
Hence, P(A) = \(\frac{m}{n}\) = \(\frac{48}{120}\) = \(\frac{2}{5}\)

Question 9.
Seven speakers A, B, C, D, E, F, G are invited in a programme to deliver speech in random order. Find the probability that speaker B delivers speech immediately after speaker A.
Answer:
Seven speakers A, B, C, D, E, F, G can deliver speech in,
n = 7P7 = 7! = 7 × 6 × 5 × 4 × 3 × 2 × 1
= 5040 ways.
A = Event that speaker B delivers speech immediately after speaker A.
The favourable outcomes for the event A are obtained as follow:
All 7 speakers can deliver speech in random in the following manners:
- If A comes first, the remaining 6 speakers can deliver their speech in 6P1 ways.
- If A comes first and B at second, the remaining 5 speakers can deliver their speech in 5P1 ways.
- If C comes after A and B, the remaining 4 speakers can deliver their speech in 4P1 ways.
- If D comes after A, B and C, the remaining 3 speakers can deliver their speech in 3P1 ways.
- If E comes after A, B, C and D, the remaining 2 speakers can deliver their speech in 2P1 ways.
- If F comes after A, B, C, D and E, the speaker G delivers his speech in 1P1 ways.
∴ Favourable outcomes for the event A is
m = 6P1 × 5P1 × 4P1 × 3P1 × 2P1 × 1P1
= 6 × 5 × 4 × 3 × 2 × 1 = 720
Hence. P(A) = \(\frac{m}{n}\) = \(\frac{720}{5040}\) = \(\frac{1}{7}\)
Alternative Method:
Seven speakers A, B, C, D, E, F, G can deliver speech in n = 7P7 = 7! = 5040 ways.
A = Event that speaker B delivers speech immediately after speaker A.
The favourable outcomes for A are obtained as follows:
Considering speakers A and B as one unit, total 6 speakers can deliver speech in 6P6 ways and B can deliver speech immediately after speaker A in 1P1 way.
∴ Favourable outcomes for A
m = 6P6 × 1P1
= 6! × 1 = 720
∴ P(A) = \(\frac{m}{n}\) = \(\frac{720}{5040}\) = \(\frac{1}{7}\)

Question 10.
Find the probability of having 5 Mondays in the month of February of a leap year.
Answer:
Total days in February of a leap year = 29
In a week there are 7 days. So in February of a leap year there are 4 weeks = 28 days and 1 day is extra.
In a week every day comes only once. Hence in 4 weeks every day comes 4 times.
∴ The sample space for 1 extra day is expressed as follows:
U = {Monday, Tuesday, Wednesday, Thursday, Friday, Saturday, Sunday}
∴ Total primary outcomes n = 7
A = Event that having 5 Mondays in the month of February in a leap year.
= {Monday}
∴ Favourable outcome for event A is m = 1
Hence, P(A) = \(\frac{m}{n}\) = \(\frac{1}{7}\)
Question 11.
Find the probability of having 53 Fridays in a -year which is not a leap year.
Answer:
A year which is not a leap year is Normal year having 365 days.
There are 52 weeks, i.e., 52 × 7 = 364 days and 1 day extra in a normal year.
So, the sample space for 1 extra day is expressed as follows:
U = {Monday, Tuesday, Wednesday, Thursday, Friday, Saturday, Sunday}
∴ Total primary outcomes n = 7
A = Event that having 53 Fridays in a year which is not a leap year.
= {Friday}
∴ Favourable outcome for the event A is m = 1
Hence, P(A) = \(\frac{m}{n}\) = \(\frac{1}{7}\)
Question 12.
Find the probability of having 5 Tuesdays in the month of August of any year.
Answer:
In the month of August there are 31 days.
4 weeks a month. So there are 4 × 7 = 28 days and 3 extra days in the month of August.
In a week each day comes only once. So in 4 weeks each day comes 4 times.
∴ The sample space for 3 extra days is expressed as follows :
U = {(Sunday, Monday, Tuesday), (Monday, Tuesday, Wednesday), (Tuesday, Wednesday, Thursday), (Wednesday, Thursday, Friday), (Thursday, Friday, Saturday), (Friday, Saturday, Sunday), (Saturday, Sunday, Monday)}
∴ Total number of primary outcomes n = 7
A = Event that having 5 Tuesdays in the month of August of any year.
= {(Sunday, Monday, Tuesday), (Monday, Tuesday, Wednesday), (Tuesday, Wednesday, Thursday)}
∴ Favourable outcomes for the event A is m = 3
Hence, P(A) = \(\frac{m}{n}\) = \(\frac{3}{7}\)

Question 13.
4 couples (husband-wife) attend a party. Two persons are randomly selected from these 8 persons. Find the probability that the selected persons are
(1) husband and wife,
(2) one man and one woman,
(3) one man and one woman who are not husband and wife.
Answer:
4 couples (husband-wife) attend a party
∴ No. of persons attending a party = 4 × 2 = 8.
Now, the number of primary outcomes of the sample space for the random experiment of selecting 2 persons randomly from 8 persons
n = 8C2 = \(\frac{8 \times 7}{2 \times 1}\) = 28
(1) A = Event that the selected two persons are husband and wife.
4 couples are there.
∴ Favourable outcomes for the event A
is m = 4C1 = 4.
Hence P(A) = \(\frac{m}{n}\) = \(\frac{4}{28}\) = \(\frac{1}{7}\)
(2) B = Event that in the selected two persons there is one man and one woman.
In 8 persons, there are 4 men and 4 women,
Favourable outcomes for the event B is
m = 4C1 × 4C1 = 4 × 4 = 16
Hence, P(A) = \(\frac{m}{n}\) = \(\frac{16}{28}\) = \(\frac{4}{7}\)
(3) C = Event that in the selected two persons there is one man and one woman who are not husband and wife.
Let 4 couples be denoted by M1F1, M2F2, M3F3, M4F4 In selected two persons if a man is selected from a couple and a woman is selected from the remaining couples, then they are not husband and wife.
∴ C = {M1F2, M1F3, M1F4, M2F1, M2F3, M2F4, M3F1, M3F2, M3F4, M4F1, M4F2, M4F3}
∴ Favourable outcomes for the event C is m = 12
Hence, P(C) = \(\frac{m}{n}\) = \(\frac{12}{28}\) = \(\frac{3}{7}\)
OR
C = B – A and A ⊂ B
∴ P(C) = P(B) – P(A) = \(\frac{4}{7}\) – \(\frac{1}{7}\) = \(\frac{3}{7}\)

Question 14.
8 workers are employed in a factory and of them are excellent in efficiency where as the rest of them are moderate in efficiency. 2 workers are randomly selected from these 8 workers. Find the probability that
(1) both the workers have excellent efficiency
(2) both the workers have moderate efficiency
(3) one worker is excellent and one worker is moderate in efficiency.
Answer:
From 8 workers 3 workers are excellent in efficiency. So the remaining 5 workers are moderate in efficiency. Total number of primary outcomes of selecting 2 workers at random from 8 workers is n = 8C2 = \(\frac{8 \times 7}{2 \times 1}\) = 28
(1) A = Event that the selected both the workers have excellent efficiency
∴ Favourable outcomes for the event A is
m = 3C2 × 5C2 = 3 × 1 = 3.
Hence, P(A) = \(\frac{m}{n}\) = \(\frac{3}{28}\)
(2) B = Event that the selected both the workers have moderate efficiency
∴ Favourable outcomes for the event B is m = 5C2 × 3C0 = 10 × 1 = 10.
Hence, P(B) = \(\frac{m}{n}\) = \(\frac{10}{28}\) = \(\frac{5}{14}\)
(3) C = Event that in the selected two workers one worker is excellent and ope worker is moderate in efficiency.
∴ Favourable outcomes for the event C is
m = 3C1 × 5C1 = 3 × 5 = 15.
Hence. P(C) = \(\frac{m}{n}\) = \(\frac{15}{28}\)

Question 15.
Two cards are drawn from a well shuffled pace of 52 cards. Find the probability that
(1) both the cards are of different colour
(2) both the cards are face cards
(3) one of the two cards is a king.
Answer:
Total number of primary outcomes of drawing two cards from a well shuffled pack of 52 cards is
n = 52C2 = \(\frac{52 \times 51}{2 \times 1}\) = 1326
(1) A = Event that both the cards drawn are of different colours, i.e., one card is of black colour and one is of red colour.
In a pack of 52 cards, 26 cards are black and 26 cards are red.
∴ Favourable outcomes for the event A is
m = 26C1 × 26C1 = 26 × 26 = 676
Hence, P(A) = \(\frac{m}{n}\) = \(\frac{676}{1326}\) = \(\frac{26}{51}\)
(2) B = Event that both the cards are face cards. In a pack of 52 cards, 12 cards are face cards.
∴ Favourable outcomes for the event B is
m = 12C2 = \(\frac{12 \times 11}{2 \times 1}\) = 66.
Hence, p(B) = \(\frac{m}{n}\) = \(\frac{66}{1326}\) = \(\frac{11}{221}\)
(3) C = Event that one of the two cards is a king. In a pack of 52 cards, 4 cards are of king and other cards are 48.
∴ Favourable outcomes for the event C is
m = 4C1 × 48C1 = 4 × 48 = 192
Hence, P(C) = \(\frac{m}{n}\) = \(\frac{192}{1326}\) = \(\frac{32}{221}\)

Question 16.
3 bulbs are defective in a box of 10 bulbs. 2 bulbs are randomly selected from this box. These bulbs are fixed in two bulb-holders installed in a room. Find the probability that the room will be lighted after starting the electric supply.
Answer:
3 bulbs are defective in a box of 10 bulbs.
∴ 7 bulbs are non-defective.
Total number of primary outcomes of selecting 2 bulbs randomly from 10 bulbs is,
n = 10C2 = \(\frac{10 \times 9}{2 \times 1}\) = 45
A = Event that the room will be lighted after starting the electric supply.
There are two options of occurring event A:
→ If 2 bulbs are non-defective and are fixed in the first bulb holder.
OR
→ If in 2 bulbs one bulb is non-defective and one bulb Is defective and are fixed in the second bulb holder.
∴ Favourable outcomes for event A is,
m = 7C2 + 7C1 × 3C1
= \(\frac{7 \times C}{2 \times 1}\) + ( 7 × 3)
= 21 + 21 = 42
∴ p(A) = \(\frac{m}{n}\) = \(\frac{42}{45}\) = \(\frac{14}{15}\)
Question 17.
For two events A and B in the sample space of a random experiment, P (A) = 0.6, P(B) = 0.5 and P(A ∩ B) = 0.15. Find
(1) P(A’)
(2) P (B – A)
(3) P (A ∩ B’)
(4) P(A’ ∩ B]
(5) P(A’ ∪ B’)
Answer:
Here, P (A) = 0.6, P (B) = 0.5 and P(A ∩ B) = 0.15 are given.
(1) P(A’) = 1 – P(A)
= 1 – 0.6
= 0.4
(2) P(B – A) = P(B) – P(A ∩ B)
= 0.5 – 0.15
= 0.35
(3) P(A ∩ B’) = P(A) – P(A ∩ B)
= 0.6 – 0.15
= 0.45
(4) As per rules of addition of Probability,
P(A ∪ B) = P(A) + P(B) – P(A ∩ B)
= 0.6 + 0.5 – 0.15
= 0.95
P(A’ ∩ B’) = P(A ∪ B)’ = 1 -P(A ∪ B)
= 1 – 0.95
= 0.05
(5) P(A’ ∪ B’) = P(A ∩ B)’= 1 – P(A ∩ B)
= 1 – 0.15
= 0.85

Question 18.
For two events A and B in the sample space of a random experiment, P (A) = 2P(B’) = 3P (A ∩ B) = 0.6. Find the probability of difference events A – B and B – A.
Answer:
P(A’) = 2P(B’) = 3P(A ∩ B) = 0.6
∴ P(A’) = 0.6,
2P(B’) = 0.6 .
3P(A ∴ B) = 0.6
∴ P(B’) = \(\frac{0.6}{2}\) = 0.3
P(A ∩ B) = \(\frac{0.6}{3}\) = 0.2
P(A – B) = P(A ∩ B’)
= P(A) – P(A ∩ B)
= [1 -P(A’)] – P(A ∩ B)
= [1 – 0.6] – 0.2
= 0.4 – 0.2
= 0.2
P(B – A) = P(A’ ∩ B)
= P(B) – P(A ∩ B)
= [1 – P(B’)] – P(A ∩ B)
= [1 – 0.3] – 0.2
= 0.7 – 0.2
= 0.5