GSEB Solutions Class 11 Maths Chapter 5 Complex Numbers and Quadratic Equations Ex 5.1

Gujarat Board GSEB Textbook Solutions Class 11 Maths Chapter 5 Complex Numbers and Quadratic Equations Ex 5.1 Textbook Questions and Answers.

Gujarat Board Textbook Solutions Class 11 Maths Chapter 5 Complex Numbers and Quadratic Equations Ex 5.1

Express each of the complex numbers given in questions 1 to 10 in the form a + ib:
1. (5i) (- \(\frac{3}{5}\) i)
2. i⁹ + i¹⁹
3. i^-39
4. 3(7 + i7) + i(7 + i7)
5. (1 – i) – (- 1 + i6)
6. (\(\frac{1}{5}\) + i\(\frac{2}{5}\)) – (4 + i\(\frac{5}{2}\))
7. [(\(\frac{1}{3}\) + i\(\frac{7}{3}\))] + (4 + i\(\frac{1}{3}\)) – (- \(\frac{4}{3}\) + i)
8. (i – 4)⁴
9. (\(\frac{1}{3}\) + 3i)³
10. (- 2 – \(\frac{1}{3}\) i)³
Solutions to questions 1 to 10:
1. (5i) (- \(\frac{3i}{5}\)) = (- 5 × \(\frac{3}{5}\)) × (i × i)
= – 3i² = (- 3)(- 1) = 3
= a + ib, where a = 3, b = 0.

2. i⁹ + i¹⁹ = i.i⁸ + i.i¹⁸ = i(i²)⁴ + i(i²)⁹
= i(- 1)⁴ + 1(- 1)⁹ = i – i = 0.
= a + ib, where a = 0, b = 0.

3. i^-39 = \(\frac{1}{i^{39}}\) = \(\frac{1}{i.i^{38}}\) = \(\frac{1}{i\left(i^{2}\right)^{19}}\) = \(\frac{1}{i(-1)^{19}}\) = – \(\frac{1}{i}\)
= – \(\frac{1}{i}\) × \(\frac{i}{i}\) = – \(\frac{i}{i^{2}}\) = \(\frac{- i}{- 1}\) = i
= a + ib, where a = 0, b = 1.

4. 3(7 + i.7) + i(7 + i.7) = (21 + 21i) + (7i + 7i²)
= (21 + 21i) + (7i – 7) = 14 + 28i
= a + ib, where a = 14, b = 28.

5. (1 – i) – (- 1 + i.6) = (1 – i) + (1 – 6i) = 1 + 1 = – i – 6i
= 2 – 7i
= (a + ib), where a = 2, b = – 7.

6. (\(\frac{1}{5}\) + i.\(\frac{2}{5}\)) – (4 + i.\(\frac{5}{2}\)) = (\(\frac{1}{5}\) + \(\frac{2}{5}\)i) + (- 4 – \(\frac{5}{2}\)i)
= \(\frac{1}{5}\) – 4 + \(\frac{2}{5}\)i – \(\frac{5}{2}\)i = – \(\frac{19}{5}\) – (- \(\frac{2}{5}\) + \(\frac{5}{2}\))i
= – \(\frac{19}{5}\) – \(\frac{21}{10}\)i = a + ib
where a = \(\frac{- 19}{5}\) and b = \(\frac{- 21}{10}\).

7. (\(\frac{1}{3}\) + i\(\frac{7}{3}\)) + (4 + i\(\frac{1}{3}\)) – (- \(\frac{4}{3}\) + i)
= (\(\frac{1}{3}\) + \(\frac{7}{3}\)i) + (4 + \(\frac{1}{3}\)i) + (\(\frac{4}{3}\) – i)
= (\(\frac{1}{3}\) + 4 + \(\frac{4}{3}\)) + i(\(\frac{7}{3}\) + \(\frac{1}{3}\) – 1) = \(\frac{17 }{3}\) + i.\(\frac{5}{3}\)
= (a + ib), where a = \(\frac{17}{3}\), b = \(\frac{5}{3}\).

8. (1 – i)⁴ = 1 – 4i + 6i² – 4i³ + i⁴
= 1 – 4i + 6(- 1) – 4i(i²) + (i²)²
= 1 – 4i – 6 – 4i(- 1) + (- 1)²
= 1 – 4i – 6 + 4i + 1
= (1 – 6 + 1) = – 4 = (a + ib),
where a = – 4, b = 0.

9. (\(\frac{1}{3}\) + 3i)³ = (\(\frac{1}{3}\))³ + 3.(\(\frac{1}{3}\))² (3i) + 3. (\(\frac{1}{3}\))(3i)² + (3i)³
= \(\frac{1}{27}\) + i + 9(- 1) + 27i³
= \(\frac{1}{27}\) + i + 9(- 1) + 27ii²
= \(\frac{1}{27}\) + i – 9 + 27i(- 1) = \(\frac{1}{27}\) + i – 9 – 27i
= (\(\frac{1}{27}\) – 9) + (1 – 27)i
= – \(\frac{242}{27}\) = – 26i
= a + ib, where a = \(\frac{- 242}{27}\), b = – 26.

10.

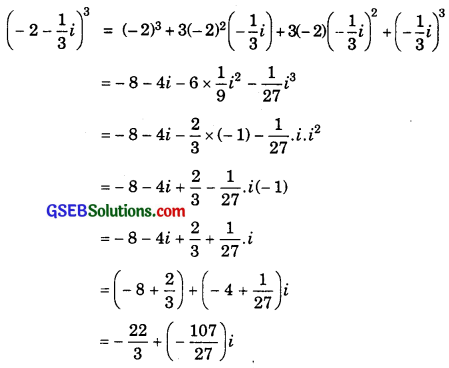
= a + ib, where a = – \(\frac{22}{3}\), b = – \(\frac{107}{27}\).

Find the multiplicative inverse of each of the complex numbers given in questions 11 to 13:
11. 4 – 3i
12. \(\sqrt{5}\) + 3i
13. – i
Solutions to questions 11 to 13:
11. Multiplicative inverse of 4 – 3i

12. Multiplicative inverse of \(\sqrt{5}\) + 3i

13. Multiplicative inverse of – i
= \(\frac{1}{- i}\) = \(\frac{- 1}{i}\) × \(\frac{i}{i}\) = \(\frac{-i}{i^{2}}\) = \(\frac{- i}{- 1}\) = i.

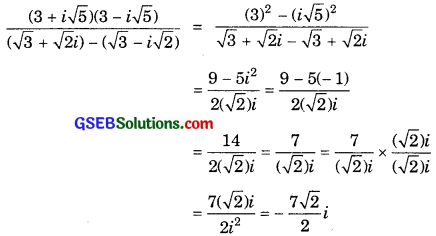
14. Express the following expression in the form a + ib:
\(\frac{(3+i \sqrt{5})(3-i \sqrt{5})}{(\sqrt{3}+\sqrt{2 i})-(\sqrt{3}-i \sqrt{2})}\)
Solution: