Gujarat Board GSEB Textbook Solutions Class 11 Maths Chapter 16 Probability Ex 16.3 Textbook Questions and Answers.
Gujarat Board Textbook Solutions Class 11 Maths Chapter 16 Probability Ex 16.3
Question 1.
Which of the following cannot be valid assignments of probability for outcomes of sample space S = {ω1, ω2, ω3, ω4, ω5, ω6, ω7}.
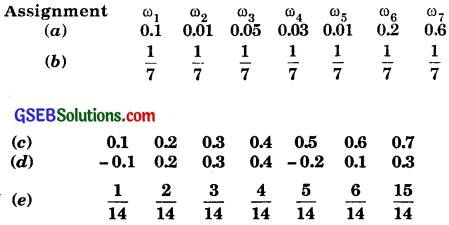
Solution:
(a) Sum of probabilities
= 0.1 + 0.01 + 0.05 + 0.03 + 0.01 + 0.2 + 0.6
= 1.00.
∴ Assignment of probabilities is valid.
(b) Sum of probabilities
= \(\frac{1}{7}\) + \(\frac{1}{7}\) + \(\frac{1}{7}\) + \(\frac{1}{7}\) + \(\frac{1}{7}\) + \(\frac{1}{7}\) + \(\frac{1}{7}\) = \(\frac{7}{7}\) = 1.
Assignment of probability is valid.
(c) Sum of probabilities
= 0.1 + 0.2 + 0.3 + 0.4 + 0.5 + 0.6 + 0.7
= 2.8
Sum of probabilities is greater than 1.
∴ This assignments of probabilities is not valid.
(d) Probability of any event cannot be negative.
Therefore, this assignment of probabilities is not valid.
(e) The last probability \(\frac{15}{14}\) is greater than 1.
∴ This assignment of probabilities is not valid.
![]()
Question 2.
A coin is tossed twice, what is the probability that at least one tail occurs?
Solution:
The sample space of the given experiment is given by
S = {HH, HT, TH, TT}
Let A be the event of getting at least one tail. Then,
A = {HT, TH, TT)
∴ P(A) = \(\frac{n(A)}{n(S)}\) = \(\frac{3}{4}\).
Question 3.
A die is thrown. Find the probability of the following events:
(i) A prime number will appear.
(ii) A number greater than or equal to 3 will appear.
(iii) A number less than or equal to 1 will appear.
(iv) A number more than 6 will appear.
(v) A number less than 6 will appear.
Solution:
The sample space associated with the experiment of throwing a die is
S = (1 ,2, 3, 4, 5, 6}.
(i) Let A be the event of having a prime number.
∴ A = {2, 3, 5}
∴ P(A) = \(\frac{n(A)}{n(S)}\) = \(\frac{3}{6}\) = \(\frac{1}{2}\).
(ii) Let A be the event of having a number ≥ 3. Then,
A = {1}.
∴ P(A) = \(\frac{n(A)}{n(S)}\) = \(\frac{4}{6}\) = \(\frac{2}{3}\).
(iii) Let A be the event of having ≤ 1. Then,
A = {1}.
∴ P(A) = \(\frac{n(A)}{n(S)}\) = \(\frac{0}{6}\) = 0.
(iv) Let A be the event of having a number more than 6. Then,
A = {1, 2, 3, 4, 5}
∴ P(A) = \(\frac{n(A)}{n(S)}\) = \(\frac{5}{6}\).
![]()
Question 4.
A card is selected from a pack of 52 cards.
(a) How many points are there in this sample space?
(b) Calculate the probability that card is an ace of spade,
(c) Calculate the probability that the card is (i) an ace (ii) black card.
Solution:
(a) There are 52 cards. Therefore, there are 52 points in the sample space.
(b) There is 1 ace of spade.
∴ n(A) = 1, n(S) = 52.
Probability that the card drawn is
an ace of spade = \(\frac{n(A)}{n(S)}\) = \(\frac{1}{52}\).
(c) (i) There are 4 aces
∴ n(A) = 4, n(S) = 52.
Probability of getting an ace card
= \(\frac{4}{52}\) = \(\frac{1}{13}\).
(ii) There are 26 black cards.
n(A) = 26, n(S) = 52.
Probability of getting a black card = \(\frac{26}{52}\) = \(\frac{1}{2}\).
![]()
Question 5.
A fair coin marked 1 on one face and 6 on the other and a fair die are both tossed. Find the probability that the sum of numbers that turn up is (i) 3 (ii) 12.
Solution:
(i) The sum is 3 when (1, 2) appear i.e., 1 on the coin and 2 on the die.
Sample space:
{(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6), (6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6)}.
∴ n(A) = 1, n(S) = 12.
∴ Probability of getting the sum 3 = \(\frac{1}{12}\).
(ii) The sum 12 will occur, when (6, 6) appears, i.e., 6 on the coin as well as 6 on the die.
∴ No. of favourable cases = 1.
∴ Probability of getting the sum 12 is \(\frac{1}{12}\).
Question 6.
There are four men and six women on the city council, If one council member is selected for a committee at random, how likely is it that it is a woman?
Solution:
There are 10 members of the council.
Any one may be selected for a committee.
∴ No. of exhaustive cases = 10.
One woman out of 6 may be selected in 6 ways.
∴ No. of favourable cases = 6.
∴ Probability of selection of a woman = \(\frac{6}{10}\) = 0.6.
![]()
Question 7.
A fair coin is tossed four times and a person wins ₹ 1 for each head and lose ₹ 1.50 for each tail that turns up.
From the sample space, calculate how many different amounts of money you can have after four tosses and the probability of having each of these amounts.
Solution:
There are five ways in which heads and tails appear.
(i) No head and 4 tails, appear
Money lost = ₹ 4 × 1.50 = ₹ 6.00
There is only 1 way when TTTT occurs.
No. of exhaustive cases = 24 = 16.
∴ Probability of getting no head or 4 tails = \(\frac{1}{16}\).
(ii) When 1 head and 3 tails appear
Money lost = ₹ (- 1 × 1 + 3 × 1.50) = ₹ 3.50.
There are 4 ways, when 1 head and 3 tails occur, i.e., HTTT, THTT, TTHT, TTTH.
Probability of getting 1 head and 3 tails = \(\frac{4}{16}\) = \(\frac{1}{4}\).
(iii) When 2 heads and 2 tails appear:
Money lost = ₹ (2 × 1.5 – 1 × 2) = ₹ (3 – 2) = ₹ 1.
2 heads and 2 tails may occur as
HHTT, HTHT, HTTH, THHT, THTH, TTHH.
Thus, 2 head and 2 tails may appear in 6 ways.
∴ Probability of getting 2 heads and 2 tails
= \(\frac{6}{16}\) = \(\frac{3}{8}\).
(iv) When 3 heads 1 tail appear
Money gained = ₹ (3 × 1 – 1 × 1.5) = ₹ 1.50.
3 heads and 1 tail may occur as HHHT, HHTH, HTHH, THHH.
∴ 3 heads and ltail appear in 4 ways
∴ Probability of gettig 3 heads and 1 tail
= \(\frac{4}{16}\) = \(\frac{1}{4}\).
(v) When all the heads appear
Money gained = ₹ 4 × 1 = ₹ 4.
4 heads occur as HHHH i.e., in one way.
∴ Probability of getting 4 heads = \(\frac{1}{16}\).
![]()
Question 8.
Three coins are tossed once. Find the probability of getting .
(i) 3 heads
(ii) 2 heads
(iii) at least 2 heads
(iv) at most 2 heads
(iv) no head
(vi) 3 tails
(vii) exactly 2 tails
(viii) no tail
(ix) at most two tails
Solution:
When three coins are tossed once, the sample space S is given by
S = {HHH, HHT, HTH, HTT, THH, THT, TTH, TTT}
Number of exhaustive cases = 8.
(i) There is only one favourable case HHH.
∴ P(3 heads) = \(\frac{1}{8}\).
(ii) There are three favourable cases, when two heads occur viz.
HHT, HTH, THH.
∴ P( exactly 2 heads) = \(\frac{3}{8}\).
(iii) At least 2 heads ⇒ 2 or 3 heads
There are 4 favourable cases HHT, HTH, THH, HHH
∴ P(at least 2 heads) = \(\frac{4}{8}\) = \(\frac{1}{2}\).
(iv) P(at most 2 heads) = P(not 3 heads)
= 1 – P(3 heads)
= 1 – \(\frac{1}{8}\) = \(\frac{7}{8}\).
(v) No head means all tails are obtained.
There is only one favourable case TTT.
∴ P(no head) = \(\frac{1}{8}\).
(vi) There is only one favourable case TTT.
∴ P(3 tails) = \(\frac{1}{8}\).
(vii) There are 3 favourable cases HTT, THT, TTH.
∴ P(exactly 2 tails) = \(\frac{3}{8}\)..
(viii) No tail means all heads are obtained.
There is only one favourable case HHH.
∴ P(all heads) = \(\frac{1}{8}\).
(ix) At most two tails ⇒ All the 3 tails do not occur 3 tails occur in 1 way.
∴ Probability that 3 tails appear = \(\frac{1}{8}\).
Probability that 3 tails do not appear = 1 – \(\frac{1}{8}\) = \(\frac{7}{8}\).
∴ Probability of getting at most two tails = \(\frac{7}{8}\).
![]()
Question 9.
If \(\frac{2}{11}\) is the probability of an event, what is the probability of the event ‘not A’.
Solution:
Let P(A) = \(\frac{2}{11}\).
∴ P(not A) = 1 – P(A) = 1 – \(\frac{2}{11}\) = \(\frac{9}{11}\).
Question 10.
A letter is chosen at random from the word ‘ASSASSINATION’. Find the probability that the letter is
(i) a vowel
(ii) a consonant.
Solution:
The word “ASSASSINATION” has 13 letters, in which there i are 6 vowels viz. AAAIIO and 7 consonants SSSSNNT.
∴ n(S) = 13.
(i) No. of vowels = 6.
∴ Probability of choosing a vowel = \(\frac{6}{13}\).
(ii) No. of consonants = 7.
∴ Probability of choosing a consonant = \(\frac{7}{13}\)
![]()
Question 11.
In a lottery, a person chooses six different natural numbers at random from 1 to 20, and if these six numbers match with the six numbers already fixed by the lot ery committee, he wins the prize. What is the probability, of winning the prize in the game?
[Hint : Order of the number is not important.]
Solution:
There are 20 natural numbers. 6 numbers may be chosen in 20C6 ways.
Now, 20C6 = \(\frac{20 \times 19 \times 18 \times 17 \times 16 \times 15}{1 \times 2 \times 3 \times 4 \times 5 \times 6}\) = 38760.
There is only 1 favourable case, which is being fixed by lottery committee.
Probability of winning the lottery = \(\frac{1}{38760}\).
Question 12.
Check whether the following probabilities P(A) and P(B) are consistent by definition:
(i) P(A) = 0.5, P(B) = 0.7, P(A ∩ B) = 0.6
(ii) P(A) = 0.5, P(B) = 0.4, P(A ∪ B) = 0.8
Solution:
(i) P(A ∩ B) must be less than or equal to P(A) and P(B).
Here, P(A ∩ B) = 0.6 > P(A)
∴ P(A) and P(B) are not consistent,
(ii) P(A ∩ B) = P(A) + P(B) – P(A ∪ B)
= 0.5 + 0.4 – 0.8
= 0.9 – 0.8
= 0.1
Thus, P(A) and P(B) are consistently defined.
![]()
Question 13.
Fill in the blanks in the following table:
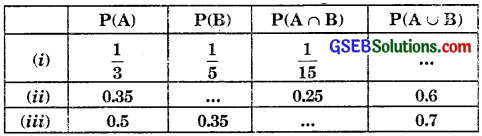
Solution:
(i) P(A ∪ B) = P(A) + P(B) – P(A ∩ B)
= \(\frac{1}{3}\) + \(\frac{1}{5}\) – \(\frac{1}{15}\) = \(\frac{5+3-1}{15}\) = \(\frac{7}{15}\).
(ii) P(A ∪ B) = P(A) + P(B) – P(A ∩ B)
0.6 = 0.35 + P(B) – 0.25
∴ P(B) = 0.6 – 0.35 + 0.25 = 0.5.
(iii) P(A ∪ B) = P(A) + P(B) – P(A ∩ B)
0.7 = 0.5 + 0.35 – P(A ∩ B)
∴ P(A ∩ B) = 0.5 + 0.35 – 0.7 = 0.15.
Question 14.
Given P(A) = \(\frac{3}{5}\) and P(B) = \(\frac{1}{5}\), find P(A or B), if A and B are mutually exclusive events.
Solution:
When A and B are mutually exclusive, then
P(A ∪ B) = P(A) + P(B)
= \(\frac{3}{5}\) + \(\frac{1}{5}\) = \(\frac{4}{5}\).
![]()
Question 15.
If E and F are events such that P(E) = \(\frac{1}{4}\), P(F) = \(\frac{1}{2}\) and P(E and F) = \(\frac{1}{8}\). Find
(i) P(E or F)
(ii) P(not E and not F).
Solution:
(i) P(E or F) = P(E ∪ F) = P(E) + P(F) – P(E ∩ F)
= \(\frac{1}{4}\) + \(\frac{1}{2}\) – \(\frac{1}{8}\)
= \(\frac{2+4-1}{8}\) = \(\frac{5}{8}\).
(ii) not E and not F = E’ ∩ F’ = (E ∪ F)’ [De Morgan’s Law]
∴ P(not E and not F) = P(E ∪ F)’
= 1 – P(E ∪ F)
= 1 – \(\frac{5}{8}\) = \(\frac{3}{8}\).
Question 16.
Events E and F are such that P (not E or not F) = 0.25. State whether E and F are mutually exclusive.
Solution:
not E or not F = E’ ∪ F’ = (E ∩ F)’
(De Morgan’s Law)
∴ P(not E or not F) = P(E ∩ F)’
= 1 – P(E ∩ F)
∴ P(E ∩ F) = 1 – 0.25 = 0.75 ≠ 0
∴ Events E and F are not mutually exclusive.
![]()
Question 17.
A and B are events such that P(A) = 0.42, P(B) = 0.48 and P(A and B) = 0.16. Determine:
(i) P (not A)
(ii) P (not B)
(iii) P (A or B).
Solution:
(i) P (not A) = P(A’) = 1 – P(A) = 1 – 0.42 = 0.58.
(ii) P (not B) = P(B’) = 1 – P(B) = 1 – 0.48 = 0.52.
(iii) P(A or B) = P(A ∪ B) = P(A) + P(B) – P(A ∩ B)
= 0.42 + 0.48 – 0.16
= 0.74.
Question 18.
In class XI of a school, 40% of the students study Mathematics and 30% study Biology and 10% of the class study both Mathematics and Biology. If a student is selected at random from the class, find the probability that he will be studying Mathematics or Biology.
Solution:
Probability that students study Mathematics
= \(\frac{40}{100}\) = 0.4.
or P(M) = 0.4.
Probability that students study Biology
= \(\frac{30}{100}\) = 0.3.
or P(B) = 0.3.
Probability that students study both Mathematics and Biology
P(M ∩ B) = 0.1.
We have to find the probability that a student studies Mathematics or Biology means we have to find P(M ∪ B)
Now, P(M or B) = P(M) + P(B) – P(M ∩ B)
= 0.4 + 0.3 – 0.1
= 0.6.
![]()
Question 19.
In an entrance test, that is graded on the basis of two examinations, the probability of a randomly chosen student passing the first examination is 0.8 and the probability of passing the second examination is 0.7. The probability of passing at least one of them is 0.95. What is the probability of passing both?
Solution:
Let A and B be the events of passing I and II examinations respectively.
P(A) = 0.8 and P(B) = 0.7.
Probability of passing at least one examination
= 1 – P(A’ ∩ B) = 0.95 ……………… (1)
Now, A’ ∩ B’ = (A ∪ B)’ [DeMorgan’s law]
So, P(A’ ∩ B’) = P(A ∪ B)’ = 1 – P(A ∪ B)
Putting this value in (1), we get
1 – [1 – P(A ∪ B)l = 0.95 or P(A ∪ B) = 0.95.
Further, P(A ∩ B) = P(A) + P(B) – P(A ∪ B)
= 0.8 + 0.7 – 0.95
= 1.5 – 0.95
= 0.55.
Thus, probability that the student will pass in both the examinations = 0.55.
Question 20.
The probability that a student will pass the final examination in both English and Hindi is 0.5 and the probability of passing neither is 0.1. If the probability of passing the English examination is 0.75, what is the probability of passing Hindi examination?
Solution:
Let E and H denote the event that a student pass in English and Hindi respectively.
The probability that a student passes in English and Hindi both is 0.1.
i.e., P(E ∩ H) = 0.5.
The probability that he passes in neither subject = 0.1.
i.e., P(E’ ∩ H’) = 0.1.
E’ ∩ H’ = (E ∪ H)’ [By De Morgan’s law]
∴ P(E’ ∩ H’) = P(E ∪ H)’ = 0.1.
or 1 – P(E ∪ H) = 0.1.
∴ P(E ∪ H) = 1 – 0.1
= 0.9.
We have, P(E ∪ H) = 0.9, P(E ∩ H) = 0.5
and P(E) = 0.75.
∴ P(E ∪ H) = P(E) + P(H) – P(E ∩ H)
0.9 = 0.75 + P(H) – 0.5.
∴ P(H) = 0.9 + 0.5 – 0.75
= 1.40 – 0.75
= 0.65.
The probability that the student will pass in Hindi is 0.65.
![]()
Question 21.
In a class of 60 students, 30 opted for NCC, 32 opted for NSS and 23 opted for both NCC and NSS. If one of these students selected at random, find the probability that
(i) The student opted for NCC or NSS,
(ii) The student has opted neither NCC nor NSS,
(iii) The student has opted NSS but not NCC.
Solution:
In a class of 60 students, 30 students opted for NCC.
∴ Probability of opting NCC = \(\frac{30}{60}\) = 0.5.
Let A be the event that a student opts NSS.
∴ P(A) = 0.5.
If B be the event that a student opts NSS.
∴ n(B) = 32.
∴ P(B) = \(\frac{32}{60}\).
24 students opt NCC and NSS both.
∴ P(A ∩ B) = \(\frac{24}{60}\).
(i) Probability that a student opts NCC or NSS
= P(A ∪ B).
Now, P(A ∪ B) = P(A) + P(B) – P(A ∩ B)
= \(\frac{30}{60}\) + \(\frac{32}{60}\) – \(\frac{24}{60}\) = \(\frac{30+32-24}{60}\)
= \(\frac{62-24}{60}\) = \(\frac{38}{60}\) = \(\frac{19}{30}\).
(ii) Probablility that the student has opted neither NCC nor NSS
= P(A’ ∩ B’)
= P(A ∪ B)’ = 1 – P(A ∪ B)
= 1 – \(\frac{19}{30}\) = \(\frac{11}{30}\).
(iii) Probability that the student has opted NSS but not NCC
= P(A’ ∩ B)
= P(B) – P(A ∩ B)
= \(\frac{32}{60}\) – \(\frac{24}{60}\)
= \(\frac{8}{60}\) = \(\frac{2}{15}\).