Gujarat Board GSEB Textbook Solutions Class 8 Maths Chapter 9 Algebraic Expressions and Identities Ex 9.2 Textbook Questions and Answers.
Gujarat Board Textbook Solutions Class 8 Maths Chapter 9 Algebraic Expressions and Identities Ex 9.2
Question 1.
Find the product of the following pairs of monomials?
- 4, 7p
- -4p, 7p
- -4p, 7pq
- 4p3, -3p
- 4p, 0
Solution:
1. 4 and 7p
4 × 7p = (4 × 7) × p = 28p
2. -4p and 7p
-4p and 7p = {(-4 × 7) × p × p = -28p2
3. -4p and 7pq
-4p × 7pq = (-4 × 7) × p × pq = -28 × p2q = -28p2q
4. 4p3 and -3p
4p3 × (-3p) = (4 × (-3)}p3 × p = -12 × p4
= -12p4
5. 4p and 0 ⇒ 4p × 0 = 0
![]()
Question 2.
Find the areas of rectangles with the following pairs of monomlaLc as their lengths and breadths respectively?
(p, q): (10m, 5n); (20x2, 5y2); (4x, 3x2); (3mn, 4np)
Solution:
(i) Length = p
Breadth = q
∴ Area of the rectangle = q = p × q = pq
(ii) Length = 10m
Breadth = 5n
∴ Area = 10m × 5n
= 10 × 5 × m × n
= 50 mn
(iii) Length = 20x2
Breadth = 5y2
∴ Area = 20x2 × 5y2
= 20 × 5 × x2 × y2
= 100x2y2
(iv) Length = 4x
Breadth = 3x2
∴ Area = 4x × 3 × x2
= 4 × 3 × x × x2 = 12x3
(v) Length = 3mn
Breadth = 4np
∴ Area = 3mn × 4np
= (3 × 4) × m × n × n × p = 12 mn2p
![]()
Question 3.
Complete the table of products?
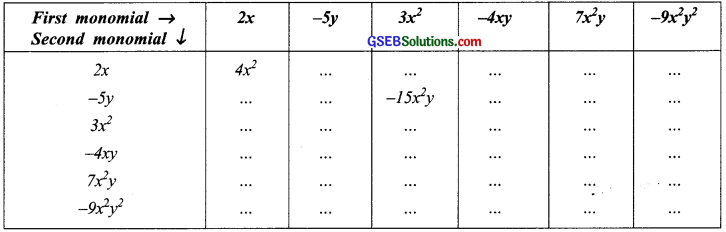 Solution:
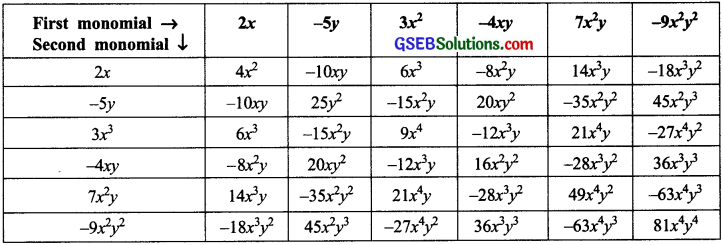
Solution:
2x × (-5y) = [2 × (-5)] × x × y = -10xy
2x × 3x2 = (2 × 3) × x × x2 = 6x3
2x × (-4xy) = [2 × (-4)] × x × xy = -8x2y
2x × 7x2y = (2 × 7) × x × x2y = 14x3y
2x × (-9x2y2) = [2 × (-9)] × x × x2y2 = -18x3y2
-5y × 2x = [-5 × 2] × y × x = -10xy
-5y × (-5y) = [-5 × 5)] × y × y = 25y2
-5y × 3x2 = (-5 × 3) × y × x2 = -15x2y
-5y × (-4xy) = [-5 × (-4)] × y × xy = 20 x2y
-5y × 7x2y = [-5 × 7] × y × x2y = -35x2y2
-5y × (-9x2y2) = [-5 × (-9)] × y × x2y2 = 45x2y3
3x2 × 2x = [3 × 2] × x2 × x = 6x3
3x2 × (-5y) = [3 × (-5)] × x2 × y = -15x2y
3x2 × 3x2 = [3 × 3] × x2 × x2 = 9x4
3x2 × (-4xy) = [3 × (-4)] × x2 × xy = -12x3y
3x2 × 7x2y = [3 × 7] × x2 × x2y
3x2 × (-9x2y2) = [3 × (-9)] × x2 × x2y2 = -27x4y2
– 4xy × 2x = [-4 × 2] × xy × x = -8x2y
– 4xy × (-5y) = [-4 × (-5)] × xy × y = 20xy2
– 4xy × 3x2 = [-4 × 3] × xy × x2 = -12x3y
– 4xy × 7x2y = [-4 × 7] × xy × x2y = -28x3y2
– 4xy × (-9x2y2) = [-4 × (-9)] × xy × x2y2 = 36x3y3
7x2y × 2x = [7 × 2] × x2y × x = 14x3y
7x2y × (-5y) = [7 × (-5)] × x2y × x = 14x3y
7x2y × (-5y) = [7 × (-5)] × x2y × y = -35x2y2
7x2y × 3x2 = [7 × 3] × x2y × x2 = 21x4y
7x2y × (-4xy) = [7 × (-4)] × x2y × xy = -28x3y2
7x2y × 7x2y = [7 × 7] × x2y × x2y = 49x4y2
7x2y × -9x2y2 = [7 × (-9)] × x2y × x2y = -63x4y3
– 9x2y2 × 2x = [-9 × 2] × x2y2 × x = -18x3y2
– 9x2y2 × (-5y) = [-9 × (-5)] × x2y2 × y = 45x2y3
– 9x2y2 × 3x2 = [-9 × 3] × x2y2 × x2 = -27x4y2
– 9x2y2 × (-4xy) = [-9 × (-4)] × x2y2 × xy = 36x3y3
– 9x2y2 × 7x2y = [-9 × 7] × x2y2 × x2y = -63x4y3
– 9x2y2 × (- 9x2y2) = [-9 × (-9)] × x2y2 × x2y2 = 81x4y4
Question 4.
Obtain the volume of rectangular boxes with the following length, breadth, height respectively?
- 5a, 3a2, 7a4
- 2p, 4q, 8r
- xy, 2x2y, 2xy2
- a, 2b, 3c
Solution:
Volume of the rectangular box
= Length × Breadth × Height
1. Length = 5a; Breadth = 3a2, Height
= 5a × 3a2 × 7a4
= (5 × 3 × 7) × a × a2 × a4
= 105 × a7 = 105a7
2. Length = 2p, Breadth = 4q, Height = 8r
∴ Volume = Length × Breadth × Height
= 2p × 4q × 8r
= (2 × 4 × 8) × p × q × r
= 64 × pqr = 64pqr
3. Length = xy, Breadth = 2x2y, Height = 2xy2
∴ Volume = Length × Breadth × Height
= xy + 2x2y × 2xy2
= (1 × 2 × 2) × xy × x2y × x2y
= 4 × x4y4 = 4x4y4
4. Length = a, Breadth = 2b, Height = 3c
∴ Volume = Length × Breadth × Height
= a × 2b × 3c
= (1 × 2 × 3) × a × b × c
= 6 × abc = 6abc
![]()
Question 5.
obtain the product of
- xy, yz, zx
- a, a -a2, a2
- 2, 4y, 8y2, 16y3
- a, 2b, 3c, 6abc
- m, -mn, mnp
Solution:
1. xy × yz × zx = (1 × 1 × 1) × x × y × y × z × z × x = 1 × (x2 × y2 × z2) = x2y2z2
2. a × (-a)2 × a3 = [1 × (-1) × 1] × a × a2 × a3
= (-1) × a6 = -a6
3. 2 × 4y × 8y2 × 16y3
= (2 × 4 × 8 × 16) × y × y2 × y3
= 1024 × y6 = 1024y6
4. a × 2b × 3c × 6abc
= (1 × 2 × 3 × 6) × a × b × c × abc
= 36 × a2b2c2 = 36a2b2c2
5. m × (-mn) × mnp = [1 × (-1) × 1] × m × mn × mnp = (-1)m2n2p = -m3n2p