GSEB Solutions Class 12 Maths Chapter 2 Inverse Trigonometric Functions Ex 2.1

Gujarat Board GSEB Textbook Solutions Class 12 Maths Chapter 2 Inverse Trigonometric Functions Ex 2.1 Textbook Questions and Answers.

Gujarat Board Textbook Solutions Class 12 Maths Chapter 2 Inverse Trigonometric Functions Ex 2.1

Find the principal values of the following:

Question 1.
sin^-1(-\(\frac { 1 }{ 2 }\))
Solution:
Let sin^-1(-\(\frac { 1 }{ 2 }\)) = y
∴ sin y = \(\frac { 1 }{ 2 }\) = – sin\(\frac { π }{ 6 }\) = sin(-\(\frac { π }{ 6 }\))
The range of principal value branch of sin^-1 is [-\(\frac { π }{ 2 }\), \(\frac { π }{ 2 }\)].
Hence, principal value of sin^-1(-\(\frac { 1 }{ 2 }\)) is – \(\frac { π }{ 6 }\).

Question 2.
cos^-1\(\left(-\frac{\sqrt{3}}{2}\right)\)
Solution:
Let cos^-1\(\left(\frac{\sqrt{3}}{2}\right)\) = y
∴ cos y = \(\left(\frac{\sqrt{3}}{2}\right)\) = – cos\(\frac { π }{ 6 }\)
The range of principal value branch is (0, π)
∴ principal value of cos^-1\(\left(\frac{\sqrt{3}}{2}\right)\) is \(\frac { π }{ 6 }\).

Question 3.
cosec^-1(2)
Solution:
Let cosec^-1(2) = y
∴ cosec y = 2 = cosec\(\frac { π }{ 6 }\)
The range of principal value branch of cosec^-1 is [-\(\frac { π }{ 2 }\), \(\frac { π }{ 2 }\)] – [0]
∴ principal value of cosec^-1(2) is \(\frac { π }{ 6 }\).

Question 4.
tan^-1(-\(\sqrt{3}\))
Solution:
Let tan^-1(-\(\sqrt{3}\)) = y
∴ tan y = 2 = – \(\sqrt{3}\) = – tan\(\frac { π }{ 3 }\) = tan(-\(\frac { π }{ 3 }\))
The range of principal value branch of tan^-1 is [-\(\frac { π }{ 2 }\), \(\frac { π }{ 2 }\)]
∴ principal value of
tan^-1(-\(\sqrt{3}\)) is – \(\frac { π }{ 3 }\).

Question 5.
cos^-1(-\(\frac { 1 }{ 2 }\))
Solution:
Let cos^-1(-\(\frac { 1 }{ 2 }\)) = y
∴ cos y = –\(\frac { 1 }{ 2 }\) = – cos\(\frac { π }{ 3 }\) = cos(π – \(\frac { π }{ 3 }\)
= cos \(\frac { 2π }{ 3 }\)
The range of principal value branch is (0, π)
∴ principal value of cos^-1(-\(\frac { 1 }{ 2 }\)) is \(\frac { 2π }{ 3 }\).

Question 6.
tan^-1(-1)
Solution:
Let tan^-1(-1) = y.
∴ tan y = – 1 = tan\(\frac { π }{ 4 }\)) = tan(\(\frac { -π }{ 4 }\))
The range of principal value branch of tan^-1 is [-\(\frac { π }{ 2 }\), \(\frac { π }{ 2 }\)]
∴ principal value of tan^-1(-1) is \(\frac { π }{4 }\).

Question 7.
sec^-1\(\left(-\frac{2}{\sqrt{3}}\right)\)
Solution:
Let sec^-1\(\left(\frac{2}{\sqrt{3}}\right)\) = y
∴ sec y = \(\left(\frac{2}{\sqrt{3}}\right)\) = sec(\(\frac { π }{ 6 }\))
The range of principal value branch of sec^-1 is [0, π], (\(\frac { π }{ 2 }\))
∴ principal value of sec^-1\(\left(\frac{2}{\sqrt{3}}\right)\) is \(\frac { π }{6 }\).

Question 8.
cot^-1(\(\sqrt{3}\))
Solution:
Let cot^-1(\(\sqrt{3}\)) = y
∴ cot y = \(\sqrt{3}\) = cot\(\frac { π }{ 6 }\)
The range of principal value branch of cot^-1 is [0, π]
∴ principal value of cot^-1\(\sqrt{3}\) is \(\frac { π }{ 6 }\).

Question 9.
cos^-1\(\left(-\frac{1}{\sqrt{2}}\right)\)
Solution:
Let cos^-1\(\left(-\frac{1}{\sqrt{2}}\right)\) = y
∴ cos y = \(\left(-\frac{1}{\sqrt{2}}\right)\) = cos(\(\frac { π }{ 4 }\)) = cos(π – \(\frac { π }{ 4 }\))
The range of principal value branch of cos^-1 is [0, π].
∴ principal value of cos^-1\(\left(-\frac{1}{\sqrt{2}}\right)\) is \(\frac { 3π }{4 }\).

Question 10.
cosec^-1 (-\(\sqrt{2}\))
Solution:
Let cosec^-1(-\(\sqrt{2}\)) = y
∴ cosec y = –\(\sqrt{2}\) = – cosec\(\frac { π }{ 4 }\) = cosec (- \(\frac { π }{ 4 }\)).
The range of principal value branch of cosec^-1 is (- \(\frac { π }{ 2 }\), \(\frac { π }{ 2 }\)) – {0}
∴ principal value of cosec^-1(-\(\sqrt{2}\)) is – \(\frac { π }{ 4 }\).

Question 11.
tan^-1(1) + cos^-1(-\(\frac { 1 }{ 2 }\)) + sin^-1(\(\frac { -1 }{ 2 }\))
Solution:
tan^-1(1) + cos^-1(-\(\frac { 1 }{ 2 }\)) + sin^-1(\(\frac { -1 }{ 2 }\))
Now, tan^-1 1 = \(\frac { π }{ 4 }\),
Since range of principal value branch of cos^-1 is [0, π]. So,

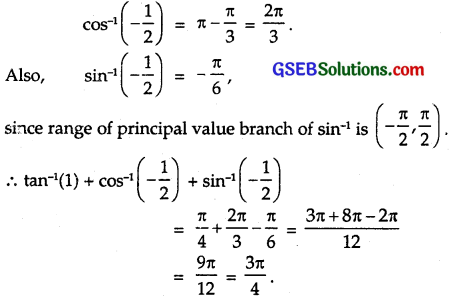
Question 12.
cos^-1(\(\frac { 1 }{ 2 }\)) + 2 sin^-1(\(\frac { 1 }{ 2 }\))
Solution:
cos^-1(\(\frac { 1 }{ 2 }\)) + 2 sin^-1(\(\frac { 1 }{ 2 }\))
Now, cos^-1(\(\frac { 1 }{ 2 }\)) = \(\frac { π }{ 3 }\)
and sin^-1(\(\frac { 1 }{ 2 }\)) = \(\frac { π }{ 6 }\)
∴ cos^-1(\(\frac { 1 }{ 2 }\)) + 2 sin^-1(\(\frac { 1 }{ 2 }\))
= \(\frac { π }{ 3 }\) + 2 x \(\frac { π }{ 6 }\) = \(\frac { 2π }{ 3 }\).

Question 13.
If sin^-1 = y, then
(A) 0 ≤ y ≤ π
(B) – \(\frac { π }{ 2 }\) ≤ y ≤ \(\frac { π }{ 2 }\)
(C) 0 < y < π
(D) – \(\frac { π }{ 2 }\) < y \(\frac { π }{ 2 }\)
Solution:
The range of principal value branch of sin^-1 is [-\(\frac { π }{ 2 }\), \(\frac { π }{ 2 }\)]
∴ if sin^-1 x = y, then – \(\frac { π }{ 2 }\) ≤ y ≤ \(\frac { π }{ 2 }\)
∴ Part (B) is correct.

Question 14.
tan^-1\(\sqrt{3}\) – sec^-1(-2) is equal to
(A) π
(B) – \(\frac { π }{ 3 }\)
(C) \(\frac { π }{ 3 }\)
(D) \(\frac { 2π }{ 3 }\)
Solution:
tan^-1\(\sqrt{3}\) = \(\frac { π }{ 3 }\)
and sec^-1(-2) = π – \(\frac { π }{ 3 }\) = \(\frac { 2π }{ 3 }\),
since principal value branch of sec^-1 is [0, π] – {\(\frac { π }{ 2 }\)}
∴ tan^-1\(\sqrt{3}\) – sec^-1(-2) = \(\frac { π }{ 3 }\) – \(\frac { 2π }{ 3 }\)
= – \(\frac { π }{ 3 }\)
∴ Part (B) is correct.